So firstly, we need to isolate the y variables to be able to solve these inequalities. Firstly, add 0.5x on both sides of the first inequality and subtract 1.5x on both sides of the second inequality:
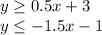
Now since the slope is positive for the first inequality, this means that the line going upwards belongs to the first inequality, and the line going downwards belongs to the second inequality.
Next, since y ≥ in the first inequality, this means that the shaded region will be above the first inequality's line, thus shading regions A and B.
Next, since y ≤ in the second inequality, this means that the shaded region is going to be below this line, thus shading regions A and C.
Since both lines shade region A, this means that region A is the solution.