Question

Simplifying Imaginary numbers

I have the answer and steps, but I have no idea how to solve it. If anyone could help please.
√-50
=i√50
=i•5√2
=5i√2
So I'm guessing the 5 and 2 is factored from 50, but how are they picked?

Answer 1

First, you must factor a -1 from the number. -50 = -1 * 50. Then you separate the roots into the product of roots. The square root of -1 is i.

`√(-50) = √(-1 * 50) = √(-1) * √(50) = i√(50)`

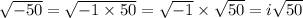
So far we took care of the negative root. Now you need to simplify the square root of 50. Since you are dealing with a square root, you need to find the largest perfect square integer that is a factor of 50. It happens to be 25. 25 is a perfect square integer since it is the square of 5, and 25 is the largest perfect square integer factor of 50 since 2 * 25 = 50. Now we factor 50 into 25 * 2, we separate the roots, and take out the root of 25.

`i√(50) = i√(25 * 2) = i√(25) * √(2) = i * 5 * √(2) = 5i√(2)`