Score 1 = 4
Score 2 = 3
Score 3 = 5
Score 1 + Score 2 + Score 3 = 12
Amy plans to give three more 5 point quizzes
Part A
Total number of quizzes Amy would have taken by the end = 6
Average score = Sum of all Scores / Total Number of quizzes
Let sum of the last 3 quizzes be x
⇒ Average Score =
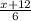
Hence, for the average to be a whole number, the sum of all her scores needs to be divisible by 6.
⇒ x+12 should be divisible by 6
Since 12 is divisible by 6, we need to make sure now that x is divisible by 6
The possible values for the scores are 0, 1, 2, 3, 4 and 5. Out of these values we will pick those 3 numbers for which the sum is a multiple of 6:
0+0+0=0
2+2+2=6
3+3+0=6
4+2+0=6
4+4+4=12
5+1+0=6
5+4+3=12
Hence, her average score would be a whole number, if she scores: 0,0,0 OR 2,2,2 OR 3,3,0 OR 4,2,0 OR 4,4,4 OR 5,1,0 OR 5,4,3
Part B
For the average to be a repeating decimal, we will definitely ignore the score combinations determined above as they lead to an average that is a whole number.
Out of 0,1,2,3,4,5, the sum of the three numbers (x) should be such that
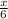 is a repeating decimal
is a repeating decimal
So if a person scores 0,0,1 then
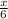 would be 0.16666.... i.e. repeating decimal (and average in this case would be
would be 0.16666.... i.e. repeating decimal (and average in this case would be
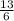 i.e. 2.166666.... , which is also a repeating decimal)
i.e. 2.166666.... , which is also a repeating decimal)
Another such combination can be 0,1,1, then
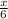 would be 0.3333..... and average in this case would be
would be 0.3333..... and average in this case would be
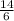 i.e. 2.33333....
i.e. 2.33333....
There can be many such combinations.