Question

the diameter of a piston for an automobile is 3 11/16in with tolerence of 1/64in. find the upper and lower limits of the pistons diameter

Answer 1

Upper Tolerance

Remark

The 11/16 is the only thing that will be affected. The three won't go up or down when we add 1/64 so we should just work with the 11/16. We need only add 11/16 and 1/64 together to see what the upper range is. Later on we can add 3 into the mix.

Solution

Upper Limit

`(11)/(16) + (1)/(64)`

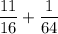
Now change the 11/16 into 64. Multiply numerator and denominator or 11/16 by 4

`(11*4)/(16*4) + (1)/(64)`

`(11*4)/(16*4) + (1)/(64)` Which results in

`(44)/(64) + (1)/(64)`

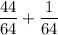
With a final result for the fractions of 45/64

So the upper tolerance = 3 45/64

Lower Tolerance

Just follow the same steps as you did for the upper tolerance except you subtract 1/64 like this.

`(11)/(16) - (1)/(64)`

Your answer should be 3 and 43/64