Question

a 40-lb container of peat moss measure 14x20x30 inches and has an average density of 0.13 g/cm^3. how many bags of peat moss are needed to cover an area measure 1 3ft by 25 ft to a depth of 1.9 inches?

Answer 1

Volume of the peat moss =
`14* 20* 30 inches`

=
`8400 in^(3)`

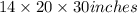
Convert the above volume into
`cm^(3)`

`1 in^(3)= 16.4 cm^(3)`

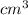
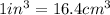
Thus, volume in
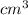`cm^(3)` is:

Volume of peat moss =
`8400 in^(3)* (16.4 cm^(3))/(1 in^(3))`

=
`137760 cm^(3)`

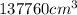
Now,

Total volume by using area and depth of the peat moss =
`area of peat moss * depth of peat moss`

=
`(13 ft *  25 ft)* 1.9 inches`

=
`(325 ft^(2))* 1.9 inches`

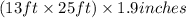
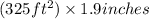
Convert above values in
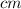`cm` to get the value of volume in
`cm^(3)`:

`1 ft= 30.48 cm`

`1 in= 2.54 cm`

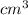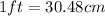
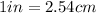
Thus, volume in
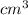`cm^(3)` is:

Total volume =
`(325 ft^(2)*((30.48 cm)^(2))/((1 ft)^(2)))* (1.9 in* (2.54 cm)/(1 in))`

=
`301934.88 cm^(2)* 4.826 cm`

=
`1457137.73088 cm^(3)`

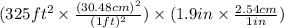
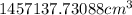
Now, number of bags is calculated by the ratio of total volume of the peat moss to the volume of the peat moss.

`Number of bags  =(total volume of peat moss)/(volume of peat moss)`

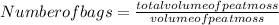
Substitute the values of volume in above formula:

`Number of bags  = (1457137.73088 cm^(3))/(137760 cm^(3))`

=
`10.57`

≅
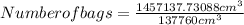
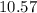
`11` bags

Thus, number of bags of peat moss are needed to cover an area measure
`13 ft` by
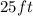`25 ft` to a depth of
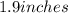`1.9 inches` are
`11` bags.