The logistic growth curve is given by the differential equation,
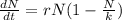
When the rate of change in population approaches the maximum carrying capacity, the curve starts to flatten or become saturated.
The left hand of the differential equation becomes zero and attains a steady state equilibrium at,
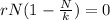
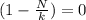
Hence, at
 .
.
The right end of the logistic growth curve shows the flattening of the curve while reaching the maximum carrying capacity.