Question

A 100 ml graduated cylinder with an inside diameter of 3.2 cm contains 34.0 g of gasoline and 34.0 g of water. what is the combined height of the two liquid layers

Answer 1

The volume of cylinder is 100 mL, inner diameter is 3.2 cm thus, radius will be:

`r=(d)/(2)=(3.2 cm)/(2)=1.6 cm`

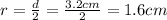
Volume of layer formed=
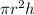`\pi r^(2)h`...... (1)

Volume of layer is also equal to sum of volume of gasoline and water.

Density of gasoline is
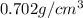`0.702 g/cm^(3)` and mass is 34 g thus, volume of gasoline will be:

`V=(m)/(d)=(34 g)/(0.702 g/cm^(3))=48.43 cm^(3)`

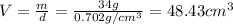
Now, density of water is
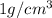`1 g/cm^(3)` and mass is 34 g thus, volume of water will be:

`V=(m)/(d)=(34 g)/(1 g/cm^(3))=34 cm^(3)`

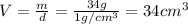
Adding both the volumes, volume of layer will be:

`Volume of layer=48.43 cm^(3)+34 cm^(3)=82.43cm^(3)`

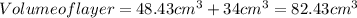
Putting the values in equation (1) to solve for height of the layer,

`h=(volume of layer)/(\pi r^(2))=(82.43 cm^(3))/(3.14* 1.6 cm* 1.6 cm)=10.25 cm`

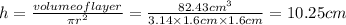
Thus, height of layer is 10.25 cm