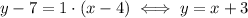Question

Write an equation in the variables x and y for the line that passes through the point (4, 7) and that is perpendicular to the line 2x + 2y = 14. provide precise expressions, not decimal approximations.

Answer 1

First of all, we need to find the slope of the given line, writing it in the
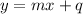`y=mx+q` form and reading the
`m` coefficient:

`2x + 2y = 14 \iff 2y = -2x + 14 \iff y = -x+7`

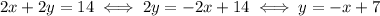
So, the slope is
`-1`

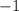
If a line with slope
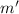`m'` is perpendicular to a line with slope
`m`, the following relationship holds:

`mm' = -1`

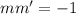
So, the slope of our line must satisfy

`m(-1) = -1 \iff m=1`

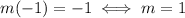
So, we need the equation of a line with slope 1 and passing through (4,7). Given the slope
`m` and a point
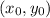`(x_0,y_0)` of a given line, its equation is given by

`y-y_0 = m(x-x_0)`

So, if you plug your values, you have

`y-7=1\cdot(x-4) \iff y = x+3`