Answer:- pH of the solution is 2.30.
Solution:- The ionization equation for the given acid is written as:
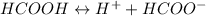
Let's say the initial concentration of the acid is c and the change in concentration x.
Then, equilibrium concentration of acid = (c-x)
and the equilibrium concentration for each of the product would be x.
Equilibrium expression for the above equation would be:
![Ka=([H^+][HCOO^-])/([HCOOH])](https://img.qammunity.org/2019/formulas/chemistry/high-school/8lgz8n1g4n77uiyr1zw71r6jnq5xoz2tt5.png)

From given info, equilibrium concentration of the acid is 0.14.
So, (c-x) = 0.14
hence,
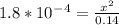
Let's solve this for x. Multiply both sides by 0.14.

taking square root to both sides:
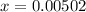
Now, we have got the concentration of
![[H^+]](https://img.qammunity.org/2019/formulas/chemistry/middle-school/cky1neqovqbkiy0bd9cgh9ska0qfqez5o0.png) .
.
![[H^+]](https://img.qammunity.org/2019/formulas/chemistry/middle-school/cky1neqovqbkiy0bd9cgh9ska0qfqez5o0.png) = 0.00502 M
= 0.00502 M
We know that,
![pH=-log[H^+]](https://img.qammunity.org/2019/formulas/chemistry/high-school/fwbsflshee2hoks5i13wxr4q8mb2gjwctk.png)
pH = -log(0.00502)
pH = 2.30
So, the pH of HCOOH solution is 2.30.