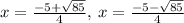Question

Solve the quadratic equation by completing the square. (enter your answers as a comma-separated list. if an answer does not exist, enter dne.) 4x2 + 10x − 15 = 0

Answer 1

`x=(-5+√(85))/(4),\:x=(-5-√(85))/(4)`

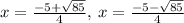
Explanation:

We are given

`4x^2+10x-15=0`

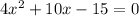
We can solve for x by completing square

Firstly, we will add both sides by 15

`4x^2+10x-15+15=0+15`

`4x^2+10x=15`

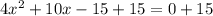
now, we can use formula

`a^2+2ab+b^2 =(a+b)^2`

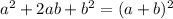
now, we can factor 10x in 2ab form

`(2x)^2+2* 2x* (5)/(2) =15`

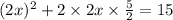
now, we can add both sides by (5/2)^2

`(2x)^2+2* 2x* (5)/(2)+((5)/(2))^2 =15+((5)/(2))^2`

so, we get square as

`(2x+(5)/(2))^2 =15+((5)/(2))^2`

`(2x+(5)/(2))^2 =(85)/(4)`

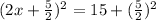
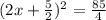
now, we can take sqrt both sides

and we get

`2x+(5)/(2)=-(√(85) )/(2) ,2x+(5)/(2)=(√(85) )/(2)`

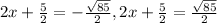
now, we can solve for x

and we get

`x=(-5+√(85))/(4),\:x=(-5-√(85))/(4)`