Question

points M,T, and D are all collinear on line segment MD. M is located at (-3,-5), T is located at (1,-3), and D is located at (9,1). What is the value for the ratio MT:MD?

Answer 1

Answer: The required ratio is MT : TD = 1 : 2.

Step-by-step explanation: Given that the points M, T and D are collinear on line segment MD.

M is located at (-3,-5), T is located at (1,-3) and D is located at (9,1).

We are to find the ratio MT : TD.

Let us consider that

MT : TD = m : n.

We know that

the co-ordinates of a point that divides a line segment with end points (a, b) and (c, d) are given by

`\left((mc+na)/(m+n),(md+nb)/(m+n)\right).`

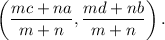
For the given situation, we have

`1=(m* 9+n* (-3))/(m+n)\\\\\\\Rightarrow m+n=9m-3n\\\\\Rightarrow 9m-m=n+3n\\\\\Rightarrow 8m=4n\\\\\Rightarrow 2m=n\\\\\Rightarrow (m)/(n)=(1)/(2)\\\\\Rightarrow m:n=1:2`

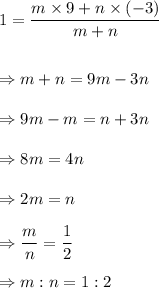
Thus, the required ratio is MT : TD = 1 : 2.

Answer 2

The ratio of interest can be found for collinear points by considering only one of the coordinates. Let's look at the x-coordinates.

(Tx -Mx)/(Dx -Mx) = (1-(-3))/(9-(-3)) = 4/12 = 1/3

Then the ratio of interest is MT:MD = 1:3.