Question

After robbing a bank in dodge city, a robber gallops off at 14 mi/h. 20 minutes later, the marshall leaves to pursue the robber at 16 mi/h. how long (in hours) does it take the marshall to catch up to the robber?

Answer 1

For this case we have to write the equation of the position of each one.

We have then:

For the robber:

`r = 14t`

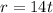
For the marshall :

`r = 16 (t- ((20)/(60)))`

`r = 16 (t-(1)/(3))`

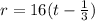
By the time the marshall reaches the robber we have:

`14t = 16 (t-(1)/(3))`

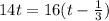
From here, we clear the time.

We have then:

`16t - 14t = (16)/(3)`

`2t = (16)/(3)`

`t =(8)/(3)`

`t = 2.7 h`

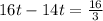
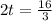
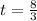
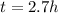
Answer:

it takes the marshall to catch up to the robber about 2.7 hours.