Question

You are to make 100.0ml of a 0.50 m acetate buffer (pka = 4.72) at ph 5.00 using 0.50 m stocks of acetic acid and sodium acetate. 6. using henderson-hasselbalch and the information given, calculate the value of [a-]/[ha].

Answer 1

`[\text{A}^(-)] / [\text{HA}] \approx 0.525`

Step-by-step explanation:

The Henderson-Hasselbalch equation estimates the value of
`[\text{A}^(-)] / [\text{HA}]` of a buffer given its
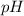`pH` and the acid dissociation constant of weak acid
`\text{HA}`:

`pH \approx pK_(a) + \text{log}_(10) ([\text{A}^(-)] / [\text{HA}])`

Rearranging:

`[\text{A}^(-)] / [\text{HA}] \approx 10^(-pH) / 10^(-pK_a)`

The question states that

• 
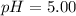  `pH = 5.00` and
• 
  `pKa = 4.72`

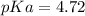
Thus

`[\text{A}^(-)] / [\text{HA}] \approx 10^(-5.00) / 10^(-4.72) = 0.525`

Reference:

Gurinder Khaira and Alexander Kot (UCD), "Henderson-Hasselbalch Approximation," Chemistry Libretext