It has been given that exactly 0.1 of the radioactive nuclei in a sample decay each hour. Thus, after n hours, the fraction of nuclei is given by the model expression:
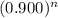 .
.
Now, to find the value of n equal to one half-life, all that we need to do is to equate the above expression to half or 0.500 (to the indicated places of decimal) and solve for n.
Thus, we will get:
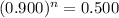
Taking the log to the base 10 on both sides, we get: (Please note that we can take the log to any base)

Dividing both sides by log(0.900), we get:
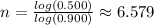 hours
hours
Therefore the value of n equal to one half-life is 6.579 hours.