Question

A roller coaster has a mass of 275 kg. It sits at the top of a hill with height 85 m. If it drops from this hill, how fast is it going when it reaches the bottom? (Assume there is no air resistance or friction.)

Answer 1

kinematic equation

v squared = u squared + 2 a x s

v= sq root (0 + 2 10 x 65)

i thimk

Answer 2

Step-by-step explanation:

Given that,

Mass of the roller costar, m = 275 kg

It sits at the top of a hill with height 85 m, h = 85 m

We need to find the speed with which it is going when it reaches the bottom. It can be calculated using the conservation of energy as :

`(1)/(2)mv^2=mgh`

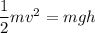
g is the acceleration due to gravity

`v=√(2gh)`

`v=√(2* 9.8* 85)`

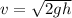
v = 40.81 m/s

So, the speed of the roller coaster when it reaches the bottom is 40.81 m/s. Hence, this is the required solution.