Answer: The required length of QS is 42 units.
Step-by-step explanation: Given that the point R is the midpoint of the line segment QS,
where QR= 8x-51 and RS = 3x-6.
We are to find the length of QS.
Since R is the midpoint of the segment QS, so we must have
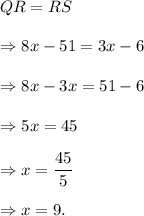
Therefore, the length of QS is given by
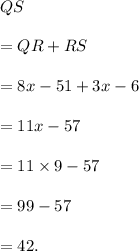
Thus, the required length of QS is 42 units.