we are given
height function as
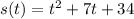
we have to find time at which height is more than 40 feet off the ground
so, we can set up inequality as
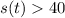
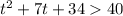
now, we can simplify it
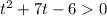
Let's assume it is equal to 0
and then we can solve for t
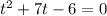
we can use quadratic formula
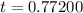
so,
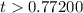
so, closest value is 1
so, captain calculus more than 40 feet off the ground after 1 seconds.......Answer