Answer:
The height of the building is 17.08 m.
Explanation:
Let the height of the building be h.
The building casts a shadow 30 m long. So, the ratio of object and its shadow is
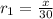
At the same time the shadow cast by a 41-cm tall pole is 72 cm long. So, the ratio of object and its shadow is
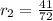
The ratio of object and its shadow is same at a point of time.


On cross multiplication we get

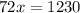
Divide both sides by 72.

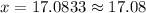
Therefore the height of the building is 17.08 m.