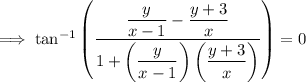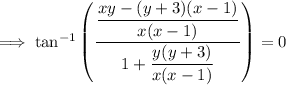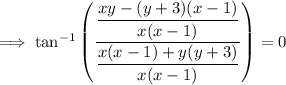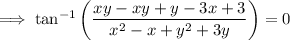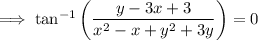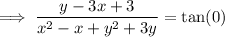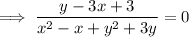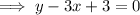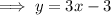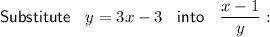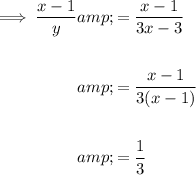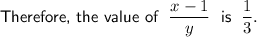Question

Please answer this question​

Answer 1

let x be the real part of z

let y be the imaginary part of z

`arg(x +i y - 1) = arg(x + iy + 3i) \\ arg(x - 1 + iy) = arg(x + (y + 3)i) \\ arctan( (y)/(x - 1) ) = arctan( (y + 3)/(x) ) \\ arctan( ( ((y)/(x - 1) ) - ( (y + 3)/(x)) )/(1 +( (y)/(x - 1) )( (y + 3)/(x) )) ) = 0 \\ arctan( ( (xy - (x - 1)(y + 3))/(x(x - 1)) )/( (x(x - 1) + y(y + 3))/(x(x - 1)) ) ) = 0`

`arctan( \frac{xy - xy - 3x + y + 3}{x {}^(2) - x + y {}^(2) + 3y } ) = 0 \\ \frac{ - 3x + y + 3}{x {}^(2) - x + y {}^(2) + 3y} = tan(0) = 0 \\ - 3x + y + 3 = 0 \\ y = 3x - 3`

`(x - 1)/(y) = (x - 1)/(3x - 3) = (x - 1)/(3(x - 1)) = (1)/(3) \\`

Answer 2

`(x-1)/(y)=(1)/(3)`

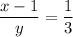
Explanation:

Sums of real and imaginary numbers are known as complex numbers:

`\large\boxed{z = x + iy}`

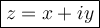
(where x is the real number and iy is the imaginary number).

Complex numbers can be represented on an Argand diagram.

• The x-axis is the real axis.
• The y-axis is the imaginary axis.

z = x + iy is represented on the diagram by the point P(x, y).

`\textsf{Argument of a complex number $= \boxed{\arg z}$}`

It is the angle between the positive real axis and the line joining that number to the origin on an Argand diagram, measured in an anticlockwise direction.

`\textsf{For \;$z = x + iy$, \;the argument $\theta$ satisfies\; $\tan \theta = (y)/(x)$.}`

`\textsf{Given: \quad $\arg (z-1)=\arg(z+3i)$}`

`\textsf{If $z$ is a complex number then $z = x + iy$}.`

`\textsf{Substitute this into the given equation}:`

`\begin{aligned} \arg(z-1)&=\arg(z+3i)\\\implies \arg(x + iy - 1) &= \arg(x + iy + 3i)\\\implies \arg((x - 1) + iy) &= \arg(x + i(y + 3))\end{aligned}`

`\textsf{If the argument $\theta$ satisfies\; $\tan \theta = (y)/(x) \implies \theta=\tan^(-1)\left((y)/(x)\right)$}:`

`\implies \tan^(-1)\left((y)/(x-1)\right)=\tan^(-1)\left((y+3)/(x)\right)`

`\implies \tan^(-1)\left((y)/(x-1)\right)-\tan^(-1)\left((y+3)/(x)\right)=0`

`\boxed{\begin{minipage}{6.2cm}\underline{Trigonometric identity}\\\\ $\tan^(-1)(a)-\tan^(-1)(b)=\tan^(-1)\left((a-b)/(1+ab)\right)$\\ \end{minipage}}`

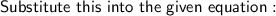
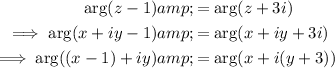
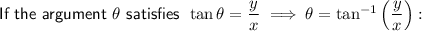
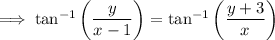
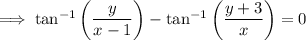
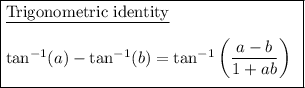
Use the arctan identity:

`\implies \tan^(-1)\left(((y)/(x-1)-(y+3)/(x))/(1+\left((y)/(x-1)\right)\left((y+3)/(x)\right)) \right)=0`

`\implies \tan^(-1)\left(((xy-(y+3)(x-1))/(x(x-1)))/(1+(y(y+3))/(x(x-1))) \right)=0`

`\implies \tan^(-1)\left(((xy-(y+3)(x-1))/(x(x-1)))/((x(x-1)+y(y+3))/(x(x-1))) \right)=0`

`\implies \tan^(-1)\left({(xy-(y+3)(x-1))/(x(x-1)+y(y+3)) \right)=0`

`\implies \tan^(-1)\left({(xy-xy+y-3x+3)/(x^2-x+y^2+3y) \right)=0`

`\implies \tan^(-1)\left({(y-3x+3)/(x^2-x+y^2+3y) \right)=0`

`\implies (y-3x+3)/(x^2-x+y^2+3y)=\tan(0)`

`\implies (y-3x+3)/(x^2-x+y^2+3y)=0`

`\implies y-3x+3=0`

`\implies y=3x-3`

`\textsf{Substitute\;\; $y=3x-3$ \;\;into \;\;$(x-1)/(y)$}:`

`\begin{aligned}\implies (x-1)/(y)& =(x-1)/(3x-3)\\\\ & =(x-1)/(3(x-1))\\\\ & =(1)/(3)\end{aligned}`

`\textsf{Therefore, the value of\; $(x-1)/(y)$\; is\; $(1)/(3)$}.`