Question

About 1.15 million people live in a circular region with a population density of about 18,075 people per square kilometer. Find the radius of the region. Round your answer to the nearest tenth.

Answer 1

Given:

Population in circular region = 1.15 million

Population Density = 18075 people per square kilometer

Formula Used:

We need to make use of the below formula to find the area of circular region.

`Area \; of \; Circular \; region \; = \; (Population)/(Density)`

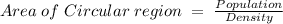
Applying the concept:

Substituting the given information in the formula above we get...

`Area \; of \; circular \; region \; = \; (1.15 * 10^6)/(18075)  \\\\Area= (1150000)/(18075)  \approx 63.6 \; km^2`

`To \; find \; radius \; of \; circle \; with \; area \; 63.6 \; square \; kilometer:\\\\Area \; of \; circle \; = \;\pi r^2\\\\\pi r^2=63.6\\\\r^2= (63.6)/(\pi)   \\\\r^2=20.25\\\\r= √(20.25)  \\\\ r \approx \; 4.5 \; km`

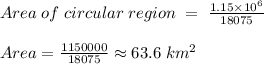
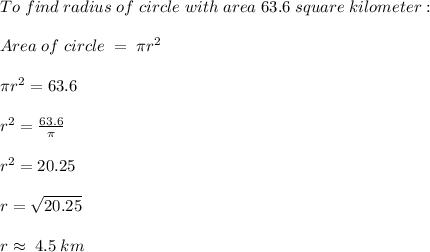
Conclusion:

The radius of the circular region rounded to the nearest tenth is 4.5 km.