Answer:
The correct option is 1.
Explanation:
The parent quadratic function is
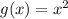
The transformation of the quadratic function is defined as
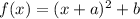 .... (1)
.... (1)
Where, a is horizontal shift and b is vertical shift.
If a>0, then the graph of function shifts a units left and if a<0, then the graph of function shifts a units right.
If b>0, then the graph of function shifts b units up and if b<0, then the graph of function shifts b units down.
It is given that the quadratic function shifted eight units to the right and one unit down. It means a=-8 and b=-1.
Substitute a=-8 and b=-1 in equation (1).
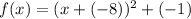

Therefore the correct option is 1.