Question

Determine whether the point (4, 9) is in the feasible set of this system of inequalities 5x + 4y <= 63 x + y <= 12 6x + 9y<= 97 x>= 0 y>= 0

Answer 1

We simply have to plug the values
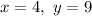`x=4,\ y=9` in all inequalities, and see if the result is true for all inequalities in the system:

`\begin{cases} 5x + 4y \leq 63 \to 5\cdot 2 + 4\cdot 9 \leq 63 \to 20+36 \leq 63\\ x + y \leq 12 \to 4+9 \leq 12 \\ 6x + 9y \leq 97 \to 6\cdot 4 + 9\cdot 9 \leq 97 \to 24+81 \leq 97 \end{cases}`

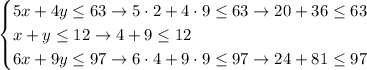
So, the system becomes

`\begin{cases} 56 \leq 63\\ 13 \leq 12 \\ 105 \leq 97 \end{cases}`

So, only the first inequality is true, and thus the point is not in the feasible set of this system of inequalities