Question

A car moving at constant speed in a straight line with the engine providing a driving force equal to the resistive force F.

When the engine is switched off, the car is brought to rest in a distance of 100 m by the resistive force.

It may be assumed that F is constant during the deceleration.

The process is then repeated for the same car with the same initial speed but with a constant resistive force of 0.800 F.

How far will the car travel while decelerating?

A. 120 m
B. 125 m
C. 156 m
D. 250 m

Answer 1

Option (b)

Step-by-step explanation:

Let the initial speed of the car is u and the final speed is zero. Let mass of the car is m.

Case I

Acceleartion of the car, a = - F/m

Use III equation of motion,

`v^(2) = u^(2) + 2 a s`

`0^(2) = u^(2) - 2 (F)/(m) * 100`

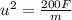`u^(2) = (200 F)/(m)` ...... (1)

Case II

Acceleration of the car, a = - 0.8 F/m

Use III equation of motion,

`v^(2) = u^(2) + 2 a s`

`0^(2) = u^(2) - 2 (0.8F)/(m) * s`

`0^(2) = u^(2) - 2 (0.8F)/(m) * s`

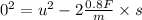
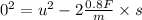
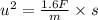`u^(2) = (1.6F)/(m) * s` ..... (2)

Dividing equation (2) by equation (1), we get

s = 125 m