Given that the revenue R (in thousands dollars) for a business is modeled by the equation
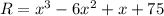
Where x represents the number of years since 2000.
Cost C (in thousands dollars) for this business is modeled by the equation

Now question is asking for the profit equation.
We know that profit occurs when Revenue is more than the cost.
So basically profit is the difference of "revenue" and "cost"
Profit P = R - C

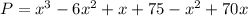
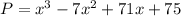
Hence final answer is
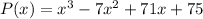 .
.