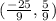The given inequality is
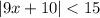
First we need to remove the absolute sign , and when we do so , we will get a compound inequality, that is

To solve for x, first we need to get rid of 10. and for that, we have to do subtraction
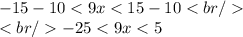
Now we need to get rid of 9, and for that, we do division
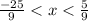
So the required solution is