Question

At the movie theatre, child admission is $5.20 and adult admission is $9.00 . On Saturday, 156 tickets were sold for a total sales of $1027.80 . How many adult tickets were sold that day?

Answer 1

We can solve this by using systems of equations.

Let's find our first formula, how much money was made using the tickets.

`5.20x + 9y = 1027.80`

Here x is how many child tickets we sold and y is how many adult tickets we sold. Now that we have defined that, we can make another formula for the total tickets sold!

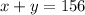`x + y = 156` since we sold 156 tickets that could be any combination of child and adult tickets.

Let's solve this system. I'm going to use substitution so I'm going to take our second formula and subtract both sides by x to get
`y = 156 - x`.

Now I will plug this in the first equation for y to get You plug it in for y to get
`5.20x + 9 (156-x) = 1027.8.`

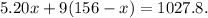
From this you can solve for x to get
`x = 99`.

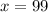Since
`x + y = 156`

`99 + y = 156`

`y = 57`

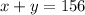
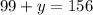
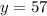
There were 99 child tickets and 57 adult tickets.