Question

What is:

`\sqrt{4x^(2) -20x+25`

Answer 1

We are given :

`\sqrt{4x^(2)-20x+25}`

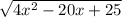
Step 1: factor the part inside square root

The function given inside square root is of quadratic form.

So let us try to factorise it using AC method.

Here A*C = 4*25 = 100

so we have to find factors of 100 that add up to give -20.

the two factors are -10 and -10.

Rewriting the function :

`4x^(2) -20x+25`

=
`4x^(2) -10x-10x+25`

=
`2x(2x-5) - 5(2x-5)`

=
`(2x-5)^(2)`

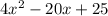
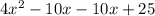
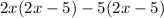
Step 2:

Now we take square root of the factorised form

`√((2x-5)^2)`

=
`2x-5`

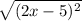
Answer : (2x-5)