Question

Q. Derive the equation of the locus of a point twice as far from (-2, 3, 4) as from (3, -1, -2)

Answer 1

The locus of all points k units away from (3, -1, -2) is the sphere

`(x-3)^2+(y+1)^2+(z+2)^2 = k^2`

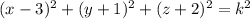
The locus of all points 2k units away from (-2, 3, 4) is the sphere

`(x+2)^2+(y-3)^2+(z-4)^2 = 4k^2`

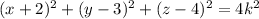
So, all points in the intersection of these two spheres are k units away from (3, -1, -2) and 2k units away from (-2, 3, 4), and thus they are twice as far from (-2, 3, 4) as from (3, -1, -2), as required.

If we divide the second equation by 4, we have

`\begin{cases} (x-3)^2+(y+1)^2+(z+2)^2 = k^2 \\ \cfrac{(x+2)^2+(y-3)^2+(z-4)^2}{4} = k^2 \end{cases}`

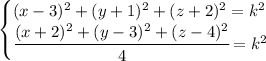
Since both left sides equal
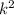`k^2`, they must be equal to each other:

`(x-3)^2+(y+1)^2+(z+2)^2 = \cfrac{(x+2)^2+(y-3)^2+(z-4)^2}{4}`

This equation describes the locus you're looking for.