In every right triangle, legs are hypothenuse are linked by the following relationship: the sine of an angle is given by the ratio of the opposite leg and the hypothenuse, while the cosine of an angle is given by the ratio of the adjacent leg and the hypothenuse.
In this case, the hypothenuse is 391 units long, while the 267-units-leg is adjacent to the angle you're interested in.
For this reason, you have

To find the value of the angle, simply invert this formula:
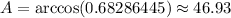
If we need to approximate this value to the nearest degree, we can observe that 46.93 lies between 46 and 47. So, we have to choose the closest one.
46 is 0.93 units far, while 47 is only 0.07 units far, so the answer is 47.