Answer:
The measure of QS is 68 units.
Explanation:
Given information: R is midpoint of QS, RS=2x-4, ST=4x-1, and RT=8x-43.
We need to find the value of QS.
From the given figure it is clear that points Q, R, S and T are collinear.
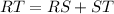
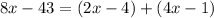
On combining like terms.

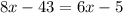
Isolate variable terms.
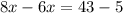
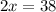
Divide both sides by 2.

The value of x is 19.
It is given that R is midpoint of QS. It means R divides the line QS in two equal parts.
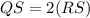
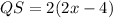
Substitute x=19 in the above equation.

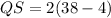
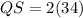
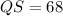
Therefore the measure of QS is 68 units.