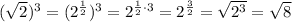
√8 is between 2 and 3, because 2²=4<8, but 3²=9>8. Also, our value is closer to 3 than to 2, so it is more than 2.5 and we have C and D options left.
Among these two numbers we find the one which is closer to √8.
C. 27=√729 ⇒ 2.7=√7.29
D. 28=√784 ⇒ 2.8=√7.84
Hence our answer is D) 2.8