Question

A blimp is 1300 meters high in the air and measures the angles of depression to two stadiums to the west of the blimp. If those measurements are 71.9 and 27.1, how far apart are the two stadiums to the nearest meter?

Answer 1

check the picture below.

the angle of depression is the angle made from the horizontal downwards, however we can use the alternate interior angles which are the angles of elevation, as you see there, since they're twins.

anyhow, if you plug that in your calculator, will give us roughly 2115.5172539029795085623.

make sure your calculator is in Degree mode.

Answer 2

2115.52 meters.

Step by step explanation:

We have been given a blimp is 1300 meters high in the air and measures the angles of depression to two stadiums to the west of the blimp. We are asked to find the distance between both stadiums.

First of all, we will find the distance between 1st stadium and base of blimp. We can see that stadium and blimp forms a right triangle with respect to ground, where m is adjacent side and 1300 meters is opposite side for 71.9 degrees angle. So we can set an equation as:

`\text{tan}=\frac{\text{Opposite}}{\text{Adjacent}}`

`\text{tan}(71.9^(\circ))=(1300)/(m)`

`m=\frac{1300}{\text{tan}(71.9^(\circ))}`

`m=(1300)/(3.059503847001)`

`m=424.905496`

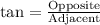
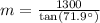
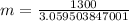
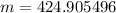
Similarly, we will find value of x as:

`\text{tan}(27.1^(\circ))=(1300)/(m+x)`

`m+x=\frac{1300}{\text{tan}(27.1^(\circ))}`

`424.905496+x=\frac{1300}{\text{tan}(27.1^(\circ))}`

`424.905496+x=2540.422750`

`424.905496-424.905496+x=2540.422750-424.905496`

`x=2115.517254`

`x\approx 2115.52`

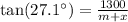
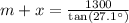
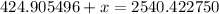
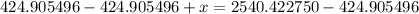
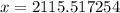
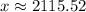
Therefore, the two stadiums are approximately 2115.52 meters apart.