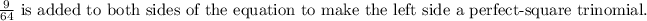Question

Brian is solving the equation x^2 -3/4 x =5 What value must be added to both sides of the equation to make the left side a perfect-square trinomial?

A)9/64

B)9/16

C)3/4

D)9/4

Answer 1

`(9)/(64)\text{ is added to both sides of the equation to make the left side a perfect-square trinomial.}`

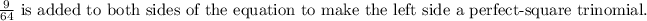
Explanation:

Given the equation

`x^2-(3)/(4)x=5`

we have to find the value which must be added to both sides of the equation to make the left side a perfect-square trinomial.

`x^2-(3)/(4)x=5`

To form the perfect square we have to add the square of half the coefficient of x,

`\text{Here the coefficient of x is }(-(3)/(4))`

`\text{Now, the square of half of above is }(-(3)/(8))^2=(9)/(64)`

`x^2-2(x)((3)/(8))+(9)/(64)=5+(9)/(64)`

`x^2-2(x)((3)/(8))+(-(3)/(8))^2=5+(9)/(64)`

`(x-(3)/(8))^2=5+(9)/(64)`

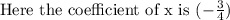
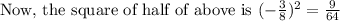
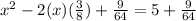
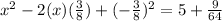
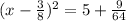
which makes LHS a perfect square trinomial.

`(9)/(64)\text{ is added to both sides of the equation to make the left side a perfect-square trinomial.}`

Answer 2

The square of half the x-coefficient must be added. That value is

... ((1/2)·(-3/4))² = (-3/8)² =

... A) 9/64