Answer:
No, (2, 0) is not a solution to the system of equations.
Explanation:
From the given graph it is notice that
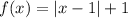

The solution of the graph is the intersection point of both functions. The graph of functions intersect each other if

The solution to the system of equations is the intersection point of both functions.
From the given graph it is noticed that the graph of both functions intersect each other at (0,2), therefore (0,2) it the solution of the system of equations.
No, (2, 0) is not a solution to the system of equations.