for a system of equations to have infinitely many solutions, it only happens when both equations are exactly equal, usually one is in disguise by some factor, like say 3x + 7 is really equals to 27x + 63, because 27x + 63 is really 9(3x + 7), so is really 3x + 7 in disguise, multiplied by 9.
anyhow, in this case we know the equations are 2(x+b) and ax + c, and we know they're exactly equal to each other, thus
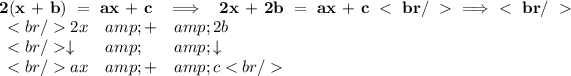
2x is the term with the variable x, ax is the term with the variable x, so 2x = ax, and 2b is just 2*b, b is a constant, so 2b is really a constant, and c is a constant as well.