It is given in the question that ,
This week his goal is to bike about 65 total miles over four days. Each day, he wants to ride 1.5 times as far as he rode the day before.
Let, on the first day, he drives x miles. On second day,he drives 1.5x. On the third day, he drives 1.5(1.5x) and on the fourth day, he drives 1.5(1.5(1.5x)).
So we have

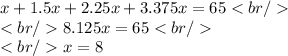
So on the first day, he drives 8 miles.
On second day, he drives 8*1.5 =12 miles
On the third day, he drives 12*1.5 = 18 miles
On the fourth day, he drives 18*1.5 = 27 miles