Question

Which is NOT a Characteristic of the linear parent function

Answer 1

I'm assuming that the "linear parent function" is
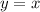`y = x`. If so:

A. is true.

In fact, the slope of a line written in the form
`y = mx+q` is
`m`, and the line
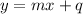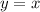`y = x` is given by
`m=1,\ q=0`

C. is true.

Since the origin is the point
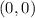`(0,0)`, if we compute the function at
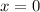`x = 0`, we want
`y = 0`. This is quite trivial since
`y = x`

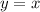
D. is true.

The domain is affected by denominators (they can be zero), even roots (they don't accept negative inputs) and logarithms (they don't accept non-positive inputs). Since this function has none of these three, the domain is all real numbers.

B. is FALSE.

The range of a function is the set of numbers obtained as output, for all possible inputs. Since, in this case, the input and the output are the same thing, if you give a negative input, you will receive the same negative output. So, the range doesn't consist only of positive numbers.

Answer 2

B-The reange is posotive real numbers