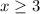Question

Solve 20x + 12 ≥ 14x + 30

Answer 1

Subtract 14x from both sides to get

`20x-14x+12 \geq 30`

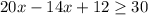
Subtract 12 from both sides to get

`20x-14x \geq 30-12`

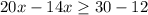
Now we have moved all terms involving x on one side, and all constant terms on the other. We can simplift both sides, i.e. sum like terms, to get

`6x \geq 18`

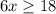
Now we have to divide both sides by 6. When dealing with inequality you have to be careful about dividing both sides by the same constant: if the constant is negative, the inequality side switches (i.e.
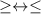`\geq \leftrightarrow \leq`). But this is not the case since 6 is positive, so we mantain the inequality sign:

on the other. We can simplift both sides, i.e. sum like terms, to get

`\cfrac{6x}{6} \geq \cfrac{18}{6}`

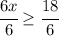
Evaluate left and right hand side:

`x \geq 3`