Question

Find BC. Round to the nearest tenth. A. 19.1 B. 20.8 C. 23.1 D. 23.3

Answer 1

m < BAC = 180 - 79 - 30 = 71 degrees

Using the Sine Rule:-

11 / sin 30 = BC / sin 71

BC = 11 * sin 71 / sin 30

= 20.8 Answer

Its choice B

Answer 2

We can use the sine rule for this problem.

Sine rule formula is:

`(a)/(Sin A) =(b)/(Sin B) =(c)/(Sin C)`

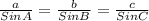
Where a (side BC), b (side AC) and c (side AB) are the opposite sides of the angle A, B and C repectively.

According to the given figure,

B= 79°, C = 30° and AB= c =11 and we need to find side BC= a.

Hence, first step is to find the angle A.

Sum of all angles of a triangle is 180°.

So, ∠A +∠B + ∠C = 180.

∠A + 79 + 30 = 180

∠A + 109 = 180

∠A = 180 - 109

∠A = 71°.

So, let's use the formula:

`(a)/(Sin A) =(c)/(Sin C)`

`(a)/(Sin 71) =(11)/(Sin 30)`

`(a)/(0.9455) =(11)/(0.5)`

`(a)/(0.9455) =22`

`(a)/(0.9455)*0.9455 =22*0.9455`

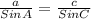
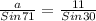
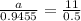
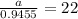
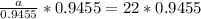
So, a = 20.801

Therefor BC is 20.8 ( Rounded to nearest tenth).

"Hope this helps!!"