This exercise wants you to build a linear system of two equations and two unknowns.
To do so, we assign a variable to each of the quantity we want to calculate, so let's call
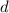 the cost of one daylily and
the cost of one daylily and
 the cost of one shrub.
the cost of one shrub.
We know that Ashley bought 7 daylilies and 2 shrubs, so she paid
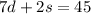
Similarly, Lisa spent
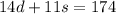
for 14 daylilies and 11 shrubs.
So, the system is
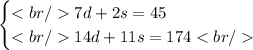
To solve the system, we must manipulate its equations in order to find the value of one particular variables. For example, we can multiply the first equation by 2 to get
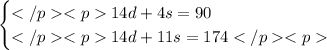
Now, if you subtract the first equation from the second you have

So, one shrub costs 12 dollars. We can substitute this value for
 in any of the equation, and solve it for
in any of the equation, and solve it for
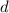 . For example, if we choose the first one we have
. For example, if we choose the first one we have

So, one daylily costs 3 dollars.