Question

Suppose a triangle has two sides of length 14 and 14, and that the angle between these two sides is 122 degrees. What is the length of the third side of the triangle?

A. 12.43
B. 18
C. 24.49
D. 22.27

Answer 1

We may use the Law of Cosines, among other methods,

`c^2 = a^2 + b^2 - 2 ab \cos C = 14^2 + 14^2 - 2(14)(14)\cos 122^\circ`

`c = √(14^2 (2 - 2 \cos 122^\circ)) = 14 √(2 - 2 \cos 122) \approx 24.48935`

Choice C

Answer 2

Suppose we have a triangle ΔABC,

Length of AB = 14

Length of AC = 14

Angle ∠BAC = 122 degree

This triangle is isosceles triangle so, angle B = angle C = (180 - 122)/2 = 29

We know the two side and one angle of triangle ABC, here we use Sine Rule.

(In trigonometry, the law of sines, sine law, sine formula, or sine rule is an equation relating the lengths of the sides of a triangle (any shape) to the sines of its angles.)

According to the law,

`(a)/(sinA) = (b)/(sinB) = (c)/(sinC)`

`(a)/(sinA) =(14)/(sin29)`

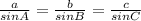
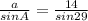
Solve for a

a = 24.49 option C