Answer:
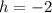
Explanation:
Parent function
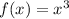
Translated function
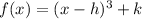
Where,
- h is the horizontal translation (x point), and
- k is the vertical translation (y point)
For the original function, the turning point is at (0,0) and for the translated function, (-2,-3) is the turning point. So x point of -2 should be in place of h, and y point of -3 should be in place of k.
Hence, value of h is -2.