Question

(EXTREMELY HARD 1000000000 POINTS)

Use the two triangles below to complete all parts to this question. Explain with as many details as possible.

You can score: 0 pt (left blank or completely wrong); 1 pt (correct answer, no explanation); 2 pts (correct answer, minimal details); 3 pts (correct answer, more than 2 details)


State whether the two figures are congruent or similar. Then provide at least two reasons how you know to support your answer. Provide as many details as you can think of.

Answer 1

• The triangles are similar, because angles are congruent and corresponding sides have same ratio.

=========================

Compare all of the corresponding parts.

Angles

• ∠A ≅ ∠D = 36°
• ∠B ≅ ∠E = 63°
• ∠C ≅ ∠F = 81°

All three angles are congruent

Sides

• AB = 5, DE = 10, DE/AB = 2
• BC = 2, EF = 4, EF/BC = 2
• AC = 4, DF = 8, DF/AC = 2

Corresponding sides have a ratio of 2.

According to our findings the triangles are similar:

• ΔABC ~ ΔDEF

Answer 2

ΔABC and ΔDEF are similar triangles as their corresponding angles are the same and their corresponding sides are in proportion.

Explanation:

Definitions

Two triangles are said to be congruent if their corresponding angles are the same and their corresponding sides are the same.

Two triangles are said to be similar if their corresponding angles are the same and their corresponding sides are in proportion.

From inspection of the given diagram, the corresponding angles of ΔABC and ΔDEF are the same, but their corresponding sides are in proportion. Therefore, they are similar triangles.

Proof of proportionality:

`\sf AB = 5, \;\;DE = 10\;\;\implies (DE)/(AB) = (10)/(5)=2`

`\sf AC = 4, \;\;DF = 8\;\; \implies (DF)/(AC) = (8)/(4)=2`

`\sf BC = 2, \;\;EF = 4\;\; \implies (EF)/(BC) = (4)/(2)=2`

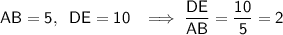
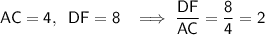
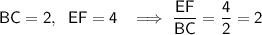
Therefore, the sides of ΔDEF are twice the length of the sides of ΔABC.