Question

A sphere with a radius of 4.8 centimeters is carved out of a right cone with a base radius of 8 centimeters and a height of 15 centimeters. What is the approximate volume of the remaining portion of the cone in terms of ?

Answer 1

Volume of the cone = 1/3 pi r^2 h = 1005.31 cm^3

Volume of the sphere = 4/3 pi r^3 = 463.25 cm^3

Volume of remaining portion of the cone = 1005.31 - 463.25

= 542.1 cm^3 to nearest tenth

Answer 2

Remaining volume of carved is 542.06 (approx 542) cm³

Explanation:

A sphere with a radius of 4.8 cm is carved out of a right cone with base of 8 cm and a height of 15 cm.

We need to find the remaining portion of the cone.

Remaining volume = Volume of cone - Volume of sphere

First we find volume of cone

Volume of cone
`=(1)/(3)* \pi * r^2* h`

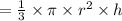
Volume of cone
`=(1)/(3)* \pi * 8^2* 15\approx 1005.31\text{ cm}^3`

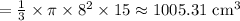
Now we find volume of sphere

Volume of sphere
`=(4)/(3)* \pi * r^3`

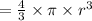
Volume of sphere
`=(4)/(3)* \pi * 4.8^3\approx 463.25\text{ cm}^3`

Remaining volume = 1005.31-463.25 = 542.06 cm³

Hence, Remaining volume of carved is 542.06 cm³