To solve this question we will make use of the basic relationship between the variables of the polar and cartesian coordinates.
They are:

and
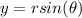 and
and
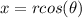
Let us solve the question now:
1.

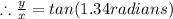
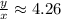
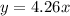
The above is the answer for question 1. It is a straight line which passes through the origin.
2. It is given that:
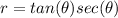
which can be rewritten as:
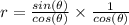
Now, we know that:
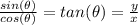
Therefore, we get:
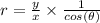
Which gives us, after cross multiplying
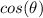 :
:
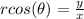
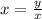 (Since,
(Since,
 )
)
Therefore,
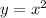 is the final answer we get by cross multiplying x.
is the final answer we get by cross multiplying x.
This is a parabola.