we are given that ,
The combined area of two squares is 17 square meters.
Area of square=
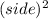
Let the side length of smaller square be x
The size of the larger Square are four times as long as the size of the smaller square
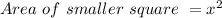
So Area of larger square=
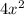
The combined Area is 17 square meter.
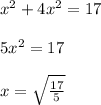
Hence the smaller square has the side length=

So the larger square has side length=
