In this question, angle 5 and angle 2 are linear pair. And angles of linear pair are supplementary angles .
Therefore
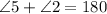
And sum of interior angles of a triangle is 180 degree, that is
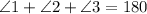
SInce both the equations have 180 in the right side, left side of both equations are equal too . That is
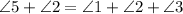
Which gives
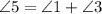
So the correct option is A .