Question

In a series circuit with three resistors connected to a power source, what would happen to the equivalent resistance and current if you removed any one of the resistors?

The equivalent resistance would decrease and the current would increase.

Answer 1

Hello! The equivalent resistance in a series circuit equals to the sum of all the resistors in the circuit.
So it’s R1+R2+R3
After u remove one of the resistors from the circuit, the equivalent becomes R1+R2, so it decreases.
The current in a series circuit will not change as the resistors change. It holds constant so there’ll be no change to the current.
Hope this helps:)

Answer 2

When three resistors are connected in series then total equivalent of all three is given by

`R_(eq)= R_1 + R_2 + R_3`

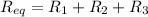
Also in this case current is given by

Now if one of the resistance is removed

Then

`R_(eq) = R_1 + R_2`

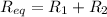
let one of the resistance is removed

while current flowing in the open circuit will be given by

`i = (V)/(R_(eq))`

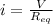
So here since resistance is decreased then current must be increased in the circuit.