Question

Posted the picture with the question so someone can help me solve

Answer 1

Since D is the midpoint of CE, knowing that CD = 3x implies that DE = 3x as well.

So far, we know that:

`AE = 2x,\quad BD = 10,\quad CE = CD = 3x`

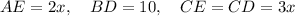
Now, since AE and BD are parallel, the two triangles ACE and BCD are similar, which means that their corresponding sides are in the same proportion.

For this reason, we can write the following proportion:

`AE : BD = CE : CD`

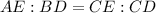
and plug the values we wrote above:

`2x: 10 = 3x : 3x`

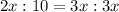
Since the two factors in the right hand side are the same, the two factors on the left hand side must be equal as well, so we deduce
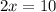`2x = 10` which solves to
`x = 5`.

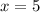Knowing that
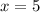`x = 5` and that
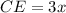`CE = 3x`, we can easily conclude that
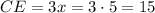`CE = 3x = 3\cdot 5 = 15`.

Answer 2

As AB=BC and ED=DC
so AE is twice of BD
AE =2BD
2x=2(10)
2x=20
Divide both sides by 2
x=10

Now CD =3x=3(10)=30

And DE=30 as D is mid point of CE
So CE =30+30=60

So CE=60
OPTION B is correct