Question

One of the lamp posts at a shopping center has a motion detector on it, and the equation (x+16)2+(y−13)2=36 describes the boundary within which motion can be sensed.

What is the greatest distance, in feet, a person could be from the lamp and be detected?

Answer 1

Answer: 6 feet is the greatest distance that a person could be from the lamp and be detected.

Explanation:

Since we have given that

The equation is :

`(x+16)^2+(y-13)^2=36`

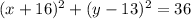
It is the equation of circle in which center is (h,k) i.e. (-16,13)

The general equation of circle is given by

`(x-h)^2+(y-k)^2=r^2`

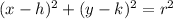
Here, the radius 'r' is given by

`r^2=36\\\\r=√(36)\\\\r=6`

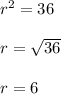
Here, the greatest distance a person could be from the lamp and be detected is represented by radius i.e. r = 6 feet.

Hence, 6 feet is the greatest distance that a person could be from the lamp and be detected.

Answer 2

(x+16)²+(y−13)²=36

The given equation is of a circle.

comparing it with general equation, radius r =6

so the lam post can detect upto 6 feet .